Amir D. Aczel The mystery of the Aleph: Mathematics, the Kasbbalah, and the Search for Infinity, Washington Square Press 2000
Unabridged Audiobook: Narrated by: Henry Leyva Length: 5 hrs and 27 mins Publisher: Random House Audio Release Date:08-20-01
2015年2月12日 木曜日 曇り時に晴れ
The essence of mathematics lies in its freedom. (G. Cantor)
Aczel The mystery of the Aleph 読了。オーディオブックをダウンロード購入したその日に全部を読んでしまった。紙の本はTy大学時代に買って読めないで今に至っていたもの。本にはさまれていた納品書をみると偶然にもちょうど3年前の2月13日の日付になっていた。私の場合、本を購入したその日に読み始めることは稀で、こんなふうにしばらく書棚にインキュベートしてからおもむろに読み始めることが多い。

The genius of Georg Cantor was that he was among the first mathmaticians (along with Bolzano, and possibly Galileo) to let his imagination run free and not be deterred by the concept of endlessness. (ibid., p140)
Cantor accepted that infinity exists even without a way to see or touch or feel every one of these endless numbers, as we seem to want to do. He could accept actual infinity rather than the safe idea of a potential infinity of the Greeks and Cantor’s contemporaries. Furthermore, Cantor was confortable with the concept that there could be different kinds of infinity: one larger than the other—something most of us find too incredible to contemplate. (ibid., p140-141)
*++**+*++*
actual infinity vs potential infinity:
Actual infinity is the idea that numbers, or some other type of mathematical object, can form an actual, completed totality; namely, a set. Hence, in the philosophy of mathematics, the abstraction of actual infinity involves the acceptance of infinite entities, such as the set of all natural numbers or an infinite sequence of rational numbers, as given objects. This is contrasted with potential infinity, in which a non-terminating process (such as “add 1 to the previous number”) produces an unending “infinite” sequence of results, but each individual result is finite and is achieved in a finite number of steps. (http://en.wikipedia.org/wiki/Actual_infinity)
***++*+**++*
本書の巻末には付録として集合論の公理が載っている。(ibid., pp229-231) 20世紀初頭に Ernst Zermelo らによってデザインされたもの。以下、名前のみ書き写しておく。
1. The Axiom of Existence:
2. The Axiom of Extension:
3. The Axiom of Specification:
4. The Axiom of Pairing:
5. The Axiom of Unions:
6. The Axiom of Powers:
7. The Axiom of Infinity:
8. The Axiom of Choice:
+*+**++*+**+
transfinite number 超限数:
Cantor called his infinities transfinite numbers, with the exception of the Absolute, which he viewed as beyond description. (ibid., p141)
transcendenbtal number 超越数:
「カントールは1874年に、実数全体の集合が非可算集合である一方で代数的数全体の集合が可算無限集合であることを示すことにより、ほとんどの実数や複素数は超越数であることを示した。」 http://ja.wikipedia.org/wiki/超越数 より引用。
「超越数(ちょうえつすう, transcendental number)とは、代数的数でない数、すなわち有理係数の代数方程式の解とならないような複素数のことである。有理数は一次方程式の解であるから、超越的な実数はすべて無理数になる。超越数論は、超越数について研究する数学の分野で、与えられた数の超越性の判定などが主な問題である。よく知られた超越数にネイピア数(自然対数の底)や円周率がある。ただし超越性が示されている実数のクラスはほんの僅かであり、与えられた数が超越数であるかどうかを調べるのは難しい問題だとされている。例えば、ネイピア数と円周率がともに超越数であることがよく知られているにもかかわらず、それをただ足しただけの π + e すら超越数かどうか分かっていない。」 http://ja.wikipedia.org/wiki/超越数 より引用。
*++*+*+*+*+*+*
axiom 公理
axioms of set theory:
The modern study of set theory was initiated by Georg Cantor and Richard Dedekind in the 1870s. After the discovery of paradoxes in naive set theory, numerous axiom systems were proposed in the early twentieth century, of which the Zermelo–Fraenkel axioms, with the axiom of choice, are the best-known. (http://en.wikipedia.org/wiki/Set_theory より引用)
Axiomatic system:
In mathematics, an axiomatic system is any set of axioms from which some or all axioms can be used in conjunction to logically derive theorems. A mathematical theory consists of an axiomatic system and all its derived theorems. An axiomatic system that is completely described is a special kind of formal system; usually though, the effort towards complete formalisation brings diminishing returns in certainty, and a lack of readability for humans. A formal theory typically means an axiomatic system, for example formulated within model theory. A formal proof is a complete rendition of a mathematical proof within a formal system. (http://en.wikipedia.org/wiki/Axiomatic_system より引用)
*****
このAczel氏のアレフ本はとてもじょうずに書かれていて、Georg Cantor から his successors Zermelo, Godel, (Cohen,) and others (ibid., 218) への学問的流れが感覚的につかめるような気持ちになりながら読み進んでゆけた。
*+*+*
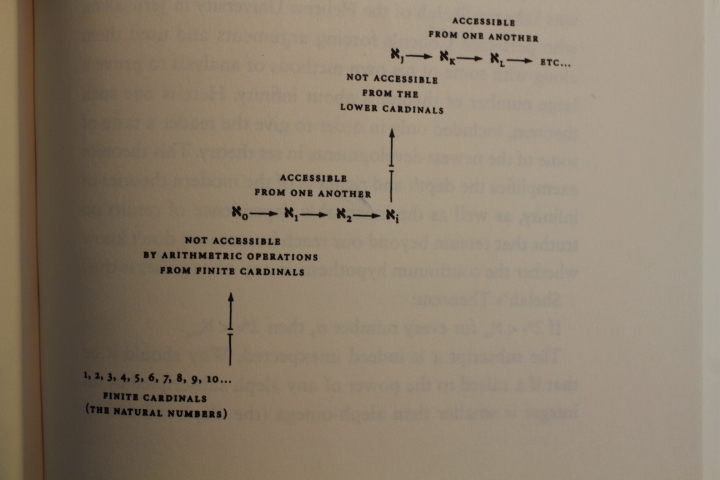
連続体仮説(れんぞくたいかせつ、Continuum Hypothesis, CH)
The Continuum Hypothesis の魅力に取り付かれた数学者の物語を読み進むうちに、あのトールキンの指輪物語の指輪に取り付かれてゆく人びとのイメージにそっくりなことに気付かされる。これはその現実数学者版。
WEBLIO辞書による簡潔な記載に依れば以下の通り http://www.weblio.jp/content/連続体仮説: 連続体仮説(れんぞくたいかせつ、Continuum Hypothesis, CH)とは、可算濃度と連続体濃度の間には他の濃度が存在しないとする仮説。19世紀にゲオルク・カントールによって提唱された。現在の数学で用いられる標準的な枠組みのもとでは「連続体仮説は証明も反証もできない命題である」ということが明確に証明されている。

*****
http://www.audible.com/pd/Bios-Memoirs/The-Mystery-of-the-Aleph-Audiobook/B002UUKQZM#publisher-summary より以下引用:
Best selling author Amir Aczel (God’s Equation) delves into the riddle of infinity, religion and science with “mad” mathematician Georg Cantor, in what Booklist calls “an indispensable book for anyone interested in the darker side of intellectual progress.”
*****
Publisher’s Summary 以下も http://www.audible.com/pd/Bios-Memoirs/The-Mystery-of-the-Aleph-Audiobook/B002UUKQZM#publisher-summary よりの引用:
“An engaging, pellucid explanation of the mathematical understanding of infinity, enlivened by a historical gloss on age-old affinities…” – Washington Post Book World
Toward the end of the 19th century, one of the most brilliant mathematicians in history languished in an asylum. His greatest accomplishment, the result of a series of extraordinary leaps of insight, was his pioneering understanding of the nature of infinity.
From the acclaimed author of God’s Equation comes The Mystery of the Aleph, the story of Georg Cantor: how he came to his theories and the reverberations of his pioneering work, the consequences of which will shape our world for the foreseeable future. The mindtwisting, deeply philosophical work of Cantor has its roots in ancient Greek mathematics and Jewish numerology as found in the mystical work known as the Kabbalah. Cantor’s theory of the infinite is famous for its many seeming contradictions; for example, we can prove that in all time there are as many years as days, that there are as many points on a one-inch line as on a one-mile line.
While the inspiration for Cantor’s mind-twisting genius lies in the very origins of mathematics, its meaning is still being interpreted. Only in 1947 did Kurt Godel prove that Cantor’s Continuum Hypothesis is independent of the rest of mathematics – and that the foundations of mathematics itself are therefore shaky.
©2001 by Amir D. Aczel; (P)2001 Random House, Inc.

*****

**********




